I agree with with you Bill but we are moving away from my point. Often it is more easier to understand the theory than to understand the difficulty of somebody else.



Let's take this obvious game

Click Here To Show Diagram Code

[go]$$W White to play
$$ -----------------------
$$ | O X . . . . . . O X |
$$ | O X . . . . . . O . |
$$ | . X . . . . . . O . |
$$ | . X . . . . . . O . |
$$ | . X . . . . . . X X |
$$ | O O . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ -----------------------[/go]

Click Here To Show Diagram Code

[go]$$W White to play
$$ -----------------------
$$ | O X . . . . . . O X |
$$ | O X . . . . . . O 6 |
$$ | 5 X . . . . . . O 4 |
$$ | 3 X . . . . . . O 2 |
$$ | 1 X . . . . . . X X |
$$ | O O . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ -----------------------[/go]

If your goal is only to get tedomari then black wins by the sequence above but it is not correct is it?
The result being not correct the rule of the game must be such that this way of playing gives the win to white.

Let's take a game for which it is time to play infinitesimals.
I agree it is not correct to play in numbers in chilled games but it is also not correct to look only for tedomari.
It seems to me that the correct interpretation stands somewhere beetween our two statements.
In chilled games, when a player passes, then it reamains only numbers.
My view is the following : the best sequence is the one which gives you the best score (the best number) and in case of equality the sequence which gives you tedomari.

With this rule, let's take again the following game

Click Here To Show Diagram Code

[go]$$W White to play
$$ -----------------------
$$ | O X . . . . . . O X |
$$ | O X . . . . . . O 6 |
$$ | 5 X . . . . . . O 4 |
$$ | 3 X . . . . . . O 2 |
$$ | 1 X . . . . . . X X |
$$ | O O . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ -----------------------[/go]

The final score is 0 and black gets tedomari

Click Here To Show Diagram Code

[go]$$W White to play
$$ -----------------------
$$ | O X . . . . . . O X |
$$ | O X . . . . . . O . |
$$ | 4 X . . . . . . O 5 |
$$ | 3 X . . . . . . O 2 |
$$ | 1 X . . . . . . X X |
$$ | O O . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ -----------------------[/go]

The final score in +1 and white gets tedomari

Due to the final score +1 this second sequence is better than first one. The score of the game has the proeminence over tedomari.

The general topic is atomic weights. I am not sure what your problem is. Or if you actually have one.



Well, in chilled go the atomic weight of the position on the right is -2, and the atomic weight on the left is +1. Why is the atomic weight +1?

I am not going to derive it, 1) because it is tedious, and 2) because you have objected to the assumptions of the derivation.

Click Here To Show Diagram Code

[go]$$W White to play
$$ -----------------------
$$ | O X . . . . . . O X |
$$ | O X . . . . . . O . |
$$ | . X . . . . . . O . |
$$ | . X . . . . . . O . |
$$ | 1 X . . . . . . X X |
$$ | O O . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ -----------------------[/go]

After the atomic weight on the left is 0. I don't know whether you agree with that or not. After all, it is a positive infinitesimal, so Black gets the last play locally, assuming correct play. The thing is, speaking informally, Black can ignore at temperature 0 in chilled go, but not White's next play there.

Click Here To Show Diagram Code

[go]$$W Sente
$$ -----------------------
$$ | O X . . . . . . O X |
$$ | O X . . . . . . O . |
$$ | 4 X . . . . . . O . |
$$ | 3 X . . . . . . O . |
$$ | 1 X . . . . . . X X |
$$ | O O . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ -----------------------[/go]

In similar manner to evaluating mean values of hot positions, atomic weight theory assumes that sente are answered, as a rule. There are execptions, OC, but that's the default.

The position on the right has atomic weight -2. That means that at temperature 0 in chilled go, White can ignore two Black plays, as a rule.

Click Here To Show Diagram Code

[go]$$B Black first
$$ -----------------------
$$ | O X . . . . . . O X |
$$ | O X . . . . . . O . |
$$ | . X . . . . . . O 3 |
$$ | . X . . . . . . O 1 |
$$ | . X . . . . . . X X |
$$ | O O . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ -----------------------[/go]

is not sente, it is ambiguous, as it does not raise the local temperature. White can ignore it. In fact, after the position on the right has atomic weight 0. It is gote, but the atomic weight is 0, even though White can reply locally.

Not that you do not know this, OC.

The atomic weight of the sum is -2 + 1 = -1. So White has the advantage in the fight for the last play at temperature 0. Getting the last play at temperature 0 is the point of atomic weight theory. That's what it is about.

Now, an advantage of 1 atomic weight is not enough to guarantee getting the last play at temperature 0, given correct play. CGT assumes correct or optimal play, unless otherwise stated. (Edit: OC, in proofs everything is stated. But the default assumption is correct or optimal play.)

Click Here To Show Diagram Code

[go]$$W Incorrect play
$$ -----------------------
$$ | O X . . . . . . O X |
$$ | O X . . . . . . O 6 |
$$ | 5 X . . . . . . O 4 |
$$ | 3 X . . . . . . O 2 |
$$ | 1 X . . . . . . X X |
$$ | O O . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ -----------------------[/go]

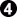
is a mistake, so this sequence lies outside the scope of atomic weight theory.

Click Here To Show Diagram Code

[go]$$W Correct play
$$ -----------------------
$$ | O X . . . . . . O X |
$$ | O X . . . . . . O . |
$$ | 4 X . . . . . . O 5 |
$$ | 3 X . . . . . . O 2 |
$$ | 1 X . . . . . . X X |
$$ | O O . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ | . . . . . . . . . . |
$$ -----------------------[/go]

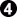
is correct, as assumed by the theory as the default. And White does get the last play at temperature 0.

_________________
The Adkins Principle:
At some point, doesn't thinking have to go on?
— Winona Adkins

Visualize whirled peas.

Everything with love. Stay safe.

My problem appear when you wrote:
The question is not that of winning the game, but of getting the last play at temperature 0

This phrasing creates some confusion when we reach the time to play infinitesimals because from this point we do not see clearly what is the relevant notion to take into account : the score to win the game, or tedomari to get the last infinitesimal?

Well I resolved my problem by creating my own solution taking into account both score and tedomari but I guess some reader may be not quite clear on this point.

The problem is really to define how to play a chilled game which is not that easy.
At the beginning it is quite simple: in order to have the right to move you must first pay a tax equal to one point. The game ends when both players passes.
Fine, but who will win the game when the two players passe?
Here the problem becomes far more difficult. Let's then assume that everybody understand(!) that all remaining areas on the board are numbers and the score of the game can be calculated just by adding these numbers.
Now I can tell who will win the game:
If the score is > 0 black wins
If the score is < 0 white wins
If the score is = 0 the player who gets tedomari wins.


You exclude as being a mistake. I agree with you OC but to be rigourous you must firstly define what means winning a chilled game. That way you can show clearly why is a mistake.
That is really my point : to show is a mistake you must take into account the score and not just tedomari.

I think a better way to have said that is that winning the game is outside the scope of the atomic weight theory. Atomic weight theory is only concerned with the question of getting the last play at temperature 0, given correct play otherwise. It is too much to ask atomic weight theory to address mistakes outside of its scope.



That's my point, as well.

_________________
The Adkins Principle:
At some point, doesn't thinking have to go on?
— Winona Adkins

Visualize whirled peas.

Everything with love. Stay safe.

Well it appears a far better way because you added "given correct play otherwise".
Now, "getting the last play at temperature 0, given correct play otherwise" isn't it equivalent to "winning the chilled game at temperature 0" as I defined it in my previous post?

Concerning "atomic weight" I built my own definition which seems to work very well. I guess it is not the definition given by the theory but I do not know what is this last one.

BTW do you know if a position equal to *4 has been discovered in go game?

Without checking, I don't think so, because you can win many chilled games without playing the infinitesimals correctly.



As I said, I have consulted the official method of calculation less often than once a year.



Nakamura found a *2 in chilled go and I found another one later on. OC, you can construct *3 by adding * and *2, but no other *3 or larger *N has been discovered, AFAIK.

_________________
The Adkins Principle:
At some point, doesn't thinking have to go on?
— Winona Adkins

Visualize whirled peas.

Everything with love. Stay safe.

It is little worrying Bill. That means that I probably miss something.
Take my definition of what is a win in a chilling game. When you compare two sequences then
1)if the score of the two sequences is different the best sequence for a player is the one which gives her the best score
2)if the score of the two sequences is equal then the best sequence for a player is the one which gives her tedomari
In my view the first case is there to avoid incorrect play and the second case is there to play correctly the infinitesimals.

IOW I hoped that this definition allows me to detect if infinetisamls are playing correctly.

Now you say that it may not be the case (?). What is your criterias to know if infinitesimals are played correctly or not?

Could you show us your *2 position you found Bill?

Even if she does not play the infinitesimals correctly.



Well, since infinitesimals occur frequently, even if they don't alway matter, I think that it is important to learn correct play that applies in general, if it exists. You learn that through difference games.

For instance, is it best for Black to play in ↓ or in {1|0||0}? Let's play the difference game. We set up the 0 game where we subtract these two from each other. Then let Black play in {1|0||0} to {1|0} and White play in {0||0|0} to {0|0}, leaving

{1|0} + {0||0|-1} + {0|0||0} + {0|0}

Black to play can win by playing to 1 in {1|0}, leaving 1 plus some infinitesimals.

If White plays in {1|0} to 0, then Black can win by playing to {0|0} in {0|0||0}, leaving {0||0|-1}, which is positive.
If White plays in {0||0|-1} to {0|-1}, then Black can win by playing to {0|0} in {0|0||0}, for a sum equal to 0.
If White plays in either of the other two games Black can win by playing to 1 in {1|0}.

So for Black to play in {1|0||0} instead of ↓ is always correct, even though ↓ has atomic weight -1.

What about the other way around? Is it better for Black to play in {0||0|-1} or in ↑? For the difference game we set up the same 0 game, but this time let Black play in {0||0|-1} and White play in {0|0||0}, leaving

{0||0|0} + {1|0||0}

Obviously, Black can win by playing to {1|0} in {1|0||0}.

White's best chance is to play to {0|0} in {0||0|0}, but then Black can win by playing to {1|0} in {1|0||0}.

So it is always correct for Black to play in {0||0|-1} instead of ↑.

OC, the ko caveat applies.

_________________
The Adkins Principle:
At some point, doesn't thinking have to go on?
— Winona Adkins

Visualize whirled peas.

Everything with love. Stay safe.

Click Here To Show Diagram Code

[go]$$ *2
$$ -------------------
$$ . . X O . . X O . .
$$ . . X X X O O O . .
$$ . . . . . . .. . .[/go]

_________________
The Adkins Principle:
At some point, doesn't thinking have to go on?
— Winona Adkins

Visualize whirled peas.

Everything with love. Stay safe.

If I understand correctly your example is equivalent to the following problem:

Click Here To Show Diagram Code

[go]$$W
$$ -----------------------
$$ | . O . . . . . . . . |
$$ | X O . . . . . . . . |
$$ | . O . . . . . . . . |
$$ | a O . . . . . . . . |
$$ | X X . . . . . . . . |
$$ | X X . . . . . . . . |
$$ | b O . . . . . . . . |
$$ | . O . . . . . . . . |
$$ | X O . . . . . . . . |
$$ -----------------------[/go]

You prove by a difference game that a black move at "a" dominates a black move at "b".
OC your example is relevant because with the chilled game I cannot distinguish between the sequence beginning by "a" and the sequence beginning by "b".
But there are no contradiction. If the chilled games cannot distinguish between the two sequences you can always try a difference game OC.

Now let me add a * to the game

Click Here To Show Diagram Code

[go]$$W
$$ -----------------------
$$ | . O X . X . . . . . |
$$ | X O O O X . . . . . |
$$ | . O . . . . . . . . |
$$ | a O . . . . . . . . |
$$ | X X . . . . . . . . |
$$ | X X . . . . . . . . |
$$ | b O . . . . . . . . |
$$ | . O . . . . . . . . |
$$ | X O . . . . . . . . |
$$ -----------------------[/go]

Now you can see clearly that the chilled game beginning by black "a" is better than the chilled game beginning by black "b" and you will be able to play correctly at "a".
Two points here:
1) A chilled game is easier to visualize in practice than a difference game (at least for me). If the chilled game is able to distinguish between two sequences then it's fine and I am happy to avoid playing a difference game
2) If the chilled game is not able to distinguish between two sequences you have two solutions:
2.1) you play at random one or the other sequence. You know that you may not play the infinitesimals in the best way but you know that for this specific game it does not harm
2.2) you try a difference game to be sure to play the infinitesimal in the best possible way. From a theoritical point of view it is far better but in practice it is not quite easy.

Fine Bill

Do you know also an *2 position for semeai where we use cooling by 2?

Well, usually difference games are homework. There are many go infinitesimals that occur again and again. These two plays, and plays like them, are common. If you know which plays are dominated, you can reduce your reading effort.



If there are many go infinitesimals on the board and one of them is unfamiliar, it may be easier to visualize a difference game between a play in it and a play in one of its rivals than to read the game out. Especially if you have experience with difference games. For instance, before reducing your first new infinitesimal, {4|*||*}, it was clear to me that it was less than ↑↑. Like ↑, it is confused with *, but it is greater than ↑. From that we can deduce that White should prefer to play in it than in ↑. In fact, in the difference game, White plays to * in one game and Black plays to * in another, so the difference game reduces to a comparison of the two games. All of this takes a matter of seconds to do in your head, if you are used to doing difference games.

_________________
The Adkins Principle:
At some point, doesn't thinking have to go on?
— Winona Adkins

Visualize whirled peas.

Everything with love. Stay safe.

Interesting Bill. I tried to generalize this:
Let's suppose G > H and G^R <= H^R where G^R and H^R are right options of G and H, then for white G^R dominates H^R.
Is it correct Bill?

You are right.

Let's work it out. We have the sum, G + H, and we want to compare a Right (White) move in G to G^R with one in H to H^R.

If H ≤ G and

G^R ≤ H^R

Then H + G^R ≤ G + H^R

I.e., in G + H, White's option, G^R + H, dominates the option, H^R + G.

_________________
The Adkins Principle:
At some point, doesn't thinking have to go on?
— Winona Adkins

Visualize whirled peas.

Everything with love. Stay safe.

Another theoritical question.
Let's take a game G and let's call L(G) and and R(G) the left stop and the right stop.
Is it possible to have L(G) < R(G) and G not a number?

Not if G is a combinatorial game.

For combinatorial games:

if L(G) < R(G) then G is a number
if L(G) = R(G) then G = L(G) or G = L(G) + an infinitesimal
if L(G) > R(G) then L(G) ≥ m(G) ≥ R(G) where m(G) is the mean value of G

_________________
The Adkins Principle:
At some point, doesn't thinking have to go on?
— Winona Adkins

Visualize whirled peas.

Everything with love. Stay safe.

OK Bill. Thank you.
BTW what is the best procedure to prove that a game is a number or not?

First, if G has no Right option or if G has no Left option, G is a number, in fact, it is an integer.

Second, if all of the Left options of G are less than all of its Right options, then G is a number.

Third, even if the first two conditions do not hold, reduce G to its simplest form, and check if they hold.

Or, as a practical matter, you can guess a number, N, and see if it is equal to G by playing G - N.

-----

Example:

{*|*} does not satisfy either of the first two conditions, but since * is an infinitesimal, if {*|*} is any number, it is 0.

Let's play {*|*} - 0 = {*|*}

If Left plays to *, White plays to 0 and wins.
If Right plays to *, Left plays to 0 and wins.

So {*|*} = 0.

-----

Is {↑*|↓*} a number? ↑* > ↓* , so we might think not. However, both ↑* and ↓* are confused with 0, so

{↑*|↓*} = 0.

_________________
The Adkins Principle:
At some point, doesn't thinking have to go on?
— Winona Adkins

Visualize whirled peas.

Everything with love. Stay safe.

Nice answer Bill. Thank you.
Guessing the number x = G seems to me a very good way of proving G is a number.
What about proving G is not a number?