This 'n' that

Bill Spight · **#541**

Thanks, John. Very interesting.

If you have any positions that seemed puzzling, if you send the references for the GoGoD sgfs we can take a look at them here.

As for outside third line contact plays, IIRC they were fairly common before the 20th century. I don't recall whether they were in the context of shimari vs. shimari, though.

John Fairbairn · **#542**

Quote:

If you have any positions that seemed puzzling

Bill: I didn't keep any record of specific games, and I mainly just looked at one side of the board using Kombilo.

However, one associated thing I noticed was that when facing shimaris occurred, often neither player seemed in a rush to grab the mid-point (to my surprise), or to be bothered by the fact the opponent got there first. What often happened (again, from memory) is that when the opponent played C10, the other player was happy to take C8. The way I rationalised this was to assume this implied two box shapes (after E10 and E8), but my analogy was to see these as pails rather than boxes. I saw the players as taking their pails to the well and carrying water back to the house.

The C10-E10 player filled his bucket to the brim and so got potentially more water, but had a big risk of spilling some and had to walk back very slowly. The C8-E8 player only filled his pail three-quarters full but didn't have to worry much about spillage and could walk back very briskly and get on first with other things.

In other words, there was some sort of quantity-quality trade-off. I'm pretty sure that applies in go, too, though I'm unsure how. But time may be an unexpected factor, though in the literal time-limit sense rather than the Go Seigen sense of getting round the board quickly. In the days of Takagawa and other big-bucket people, having up to 13 hours each to think possibly meant you could take the risk of filling a potentially leaky bucket (i.e. pre-consider all the aji and erasure possibilities). I suspect that nowadays, with shorter limits, players have become me pragmatic and are more often happy with an underfilled bucket. (Maybe the Henry and Liza bucket roles have been reversed ?

)

Here's something a bit different. It's not quite the same thing as you are discussing, but may offer a sidelight. It's from a Genjo-Chitoku game (my book is now finished and had several examples of the sort of thing that baffles me here, which is why I possibly sound "primed" on this topic).

After White 8, Black does not rush to play R10. White 10 (by Genjo) obviously has ladder implications, but still, with the subsequent moves, has an air of the sort of whole-board play and influence rather than thickness the bots are showing us, especially with 16 (which allows Black a mid-point play at 17, which, however, later commentators thought was too close to White's influence. The modesty of the tsume at 18 is instructive (because he is about to strengthen Black with 20-21. Turning away at 22 (because he cannot attack Black on the right yet) also has bottish overtones to me.

Here is another G-Ch example, though less baffling to me:

White 12 favours the "wrong" extension (less baffling because 13 and 14 can be regarded as miai). But the attempt to overconcentrate Black immediately with 16 etc is an aspect of bot play that modern Japanese players have latched on to strongly.

Bill Spight · **#543**

Thanks, John! Ah, yes, Genjo vs. Chitoku.

That 5-5 rang a bell. Cool move. :cool:

Bill Spight · **#544**

Some comments and Elf variations for the second game. Some surprising error estimates.

Edit: Added variation for :w12:

.
Edit: And another.

Bill Spight · **#545**

In the first game, both players had a blind spot about the top left corner, such that Elf's analysis had a seesaw effect, with each player losing points on almost every play for not playing in the top left corner. Still, there are some fascinating variations.

Uberdude · **#546**

re n11, I think a lot of the value comes not from its attacking element, but its defensive reverse sente aspect: denying black the pleasing haengma of running plus shape attack at n12. It's double sente.

Ignoring the peep is probably only justified because of giving komi: in a no komi game Black can probably get away with the heavier play of the game denying white easy cash (though I'd like to see how Elf uses aji of those cut stones later:the right side isn't solid cash). Komi or not though, I suspect the suggested large Knight jump on a white shape point is just better than the one point jump. Because white didn't do the close pincer Black can play thinner. (or even should to avoid inefficiency, we can anticipate shoulder hit or attachment against the 2-space pincer in which case one point jump is overconcentrated.)

The 5-4 doesn't have particularly complicated variations does it? If it was a 5-3 I could understand black not going in as "I know going in is probably the best move, but I'm scared my opponent has some secret new taisha variation to trick me so I won't go in" whilst white thinks "I don't need to close the corner because I've psyched out my opponent with my mad taisha skills". Also notable that white closed it in a bigger way than Elf recommended this game, presumably lack of komi requiring more ambitious play.

Bill Spight · **#547**

Uberdude wrote:

re n11, I think a lot of the value comes not from its attacking element, but its defensive reverse sente aspect: denying black the pleasing haengma of running plus shape attack at n12. It's double sente.

Well White N-11 takes on a defensive character after Black L-16. But without N-11 in place, I suspect that Black would approach the top left corner at G-17, as he does in at least one variation. But then if White plays a one space pincer and after Black jumps out, plays M-16, N-11 looks very nice. Perhaps that's why Elf played Black L-16. :scratch:

Quote:

Ignoring the peep is probably only justified because of giving komi: in a no komi game Black can probably get away with the heavier play of the game denying white easy cash (though I'd like to see how Elf uses aji of those cut stones later:the right side isn't solid cash).

Well, certainly Black tends to play conservatively with no komi. Such a large furikawari would be quite a bold stroke.

But if Black believed, with Elf, that the top left corner was the hottest spot on the board, getting there first would be attractive. (OC, in that case Black would have played there earlier.)

Quote:

Komi or not though, I suspect the suggested large Knight jump on a white shape point is just better than the one point jump. Because white didn't do the close pincer Black can play thinner. (or even should to avoid inefficiency, we can anticipate shoulder hit or attachment against the 2-space pincer in which case one point jump is overconcentrated.)

Yes, I don't think that Elf says much about no komi go, particularly about White's play. But, especially in the early game, I we can learn a good bit from its analysis. Most preferred plays with 7.5 komi will remain preferred with no komi, even though the win rate estimates would be different. I think that the large knight's jump versus the one space jump is a case in point.

Bill Spight · **#548**

Evaluation and Tic-Tac-Toe

Traditional go evaluation does not depend upon whose turn it is and assumes perfect play. Minimax evaluation depends upon whose turn it is and assumes perfect play. Winrate evaluation depends upon whose turn it is and assumes imperfect play (except when the winrate is 100% or 0%). OC, for Tic-Tac-Toe we know perfect play.

Lately I have been wondering how to introduce the idea of traditional go evaluation to those who know nothing about it, and the idea of using Tic-Tac-Toe came to mind. I learned a little something about Tic-Tac-Toe and I thought I would write it up.

Despite not knowing perfect play in go we can evaluate the empty board. Because of symmetry it has an average value of zero. The result with perfect play when Black plays first has to be the negative of perfect play when White plays first, and their average is zero. The same reasoning applies to Tic-Tac-Toe. Traditional go evaluation has not been very successful in making a strong go player. One reason for that is that another variable, that of temperature, is important. You can’t simply rely upon the average value of a position. Tic-Tac-Toe provides a good example of this, which I shall illustrate using go diagrams.

Click Here To Show Diagram Code: [go]$$Bc Value 0 $$ ------- $$ | . . . | $$ | . . . | $$ | . . . | $$ -------[/go]

The empty board has a value of zero and the final board also has a value of 0, counting a win for Black as +1 and a win for White as -1. It’s temperature is 0.

Click Here To Show Diagram Code: [go]$$Bc Value 0 $$ ------- $$ | . . 1 | $$ | . . . | $$ | 2 . . | $$ -------[/go]

After

takes one corner, :w2:

takes the opposite corner. By symmetry we know that this board also has an average value of 0. But it has a temperature of 1. Whoever plays first can win with perfect play.

Click Here To Show Diagram Code: [go]$$Bc Value 1 $$ ------- $$ | 5 a 1 | $$ | . b 4 | $$ | 2 . 3 | $$ -------[/go]

forces

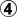
, and then :b5:

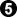
produces a won position. Even if White plays first Black has the miai of “a” and “b” to win; if White takes one, Black takes the other. The players could stop play now and declare a Black win.

When I was a kid I was unaware of this little trick, because I always made my first play in the center, whether as X or O.

It’s a good illustration of why simply knowing the traditional go value of a position is not enough to base play on. After :w1:

White may reason that :w2:

is OK because it returns the board to its original value of 0. But :w2:

raises the temperature to 1 and allows Black to win. I believe that using minimax evaluation chess programs used to (and maybe still do) evaluate only quiescent positions, for a similar reason.

Suppose we did not know perfect play for Tic-Tac-Toe. What would the winrate value of the first play? We do not know, because winrate values depend upon imperfect play, and we do not know the error functions of the players. However, I think we can say something about error rates, even without that knowledge.

Click Here To Show Diagram Code: [go]$$Bc Error $$ ------- $$ | . 1 5 | $$ | 2 3 . | $$ | . 4 . | $$ -------[/go]

is a mistake. Opening on the side gives the second player 2 potential errors out of 8 plays (1 out of 5 if you eliminate symmetrical plays.)

Click Here To Show Diagram Code: [go]$$Bc Error $$ ------- $$ | 3 . 5 | $$ | 2 1 . | $$ | . . 4 | $$ -------[/go]

is a mistake. Opening in the center gives the second player 4 potential errors out of 8 plays (1 out of 2) if you eliminate symmetrical plays.)

Click Here To Show Diagram Code: [go]$$Bc Error $$ ------- $$ | 2 . 1 | $$ | . . . | $$ | . . 3 | $$ -------[/go]

is a mistake. :b3:

transposes to a win we have already seen (by symmetry).

Click Here To Show Diagram Code: [go]$$Bc Error $$ ------- $$ | . 2 1 | $$ | . 5 4 | $$ | . . 3 | $$ -------[/go]

is a mistake.

Click Here To Show Diagram Code: [go]$$Bc Error $$ ------- $$ | 3 . 1 | $$ | 2 . . | $$ | . . . | $$ -------[/go]

is a mistake. :b3:

transposes to the win in the previous diagram.

Opening in the corner gives the second player 7 possible errors out of 8 plays (4 out of 5 if you eliminate symmetrical plays).

My guess is that a bot trained on winrate evaluation, but not to perfect play, would be quite likely to make the first play in a corner.

sorin · **#549**

Bill Spight wrote:

Evaluation and Tic-Tac-Toe

Traditional go evaluation does not depend upon whose turn it is and assumes perfect play. Minimax evaluation depends upon whose turn it is and assumes perfect play. Winrate evaluation depends upon whose turn it is and assumes imperfect play (except when the winrate is 100% or 0%). OC, for Tic-Tac-Toe we know perfect play.

Lately I have been wondering how to introduce the idea of traditional go evaluation to those who know nothing about it, and the idea of using Tic-Tac-Toe came to mind.

This reminded me about a very simple computer which can be used to implement Reinforcement Learning for Tic Tac Toe, consisting of 300 matchboxes :-)

https://www.belloflostsouls.net/2019/03 ... c-toe.html

Bill Spight · **#550**

sorin wrote:

This reminded me about a very simple computer which can be used to implement Reinforcement Learning for Tic Tac Toe, consisting of 300 matchboxes :-)

https://www.belloflostsouls.net/2019/03 ... c-toe.html

Yeah, Martin Gardner wrote about that in his column in Scientific American.

sorin · **#551**

Bill Spight wrote:

Opening in the corner gives the second player 7 possible errors out of 8 plays (4 out of 5 if you eliminate symmetrical plays).

My guess is that a bot trained on winrate evaluation, but not to perfect play, would be quite likely to make the first play in a corner.

Bill, I think there is something wrong with this conclusion. The reason I say that: a bot trained from zero will eventually find out that opening in the center results in wins more often than opening somewhere else.

Also, about "bot trained on winrate evaluation, but not to perfect play": this distinction matters only for trivial games, like Tic Tac Toe, while for any interesting games, no bot can be trained "to perfect play" :-)

Bill Spight · **#552**

sorin wrote:

Also, about "bot trained on winrate evaluation, but not to perfect play": this distinction matters only for trivial games, like Tic Tac Toe, while for any interesting games, no bot can be trained "to perfect play" :-)

Quite so.

sorin wrote:

Bill Spight wrote:

Opening in the corner gives the second player 7 possible errors out of 8 plays (4 out of 5 if you eliminate symmetrical plays).

My guess is that a bot trained on winrate evaluation, but not to perfect play, would be quite likely to make the first play in a corner.

Bill, I think there is something wrong with this conclusion. The reason I say that: a bot trained from zero will eventually find out that opening in the center results in wins more often than opening somewhere else.

The defense to the center opening is to play in a corner (of which there are four). The only defense to a play in a corner is to play in the center (only one point); the second player has no leeway.

lightvector · **#553**

Yeah, the corner opening is often better than the center opening against humans too.

Even if they respond in the center (the single non-losing reply), you can still sometimes steal a game from them if they're unfamiliar with the position like this:

Click Here To Show Diagram Code: [go]$$B $$ ------- $$ | 3 . . | $$ | . 2 . | $$ | . . 1 | $$ -------[/go]

Now, if they play one of the corners, you win. Back in early grade school, I won some games this way against people who were overconfident that they could never lose at tic-tac-toe, but who didn't actually think about their moves. Avoiding these pitfalls requires more than 1 move lookahead, whereas most (although not all) of the center-opening lines after initial reply only require the defender to further play moves to prevent an immediate win, without needing any lookahead. Along with unfamiliarity for many opponents, this makes the corner opening much stronger than the center.

sorin · **#554**

Bill Spight wrote:

sorin wrote:

Bill Spight wrote:

Opening in the corner gives the second player 7 possible errors out of 8 plays (4 out of 5 if you eliminate symmetrical plays).

My guess is that a bot trained on winrate evaluation, but not to perfect play, would be quite likely to make the first play in a corner.

Bill, I think there is something wrong with this conclusion. The reason I say that: a bot trained from zero will eventually find out that opening in the center results in wins more often than opening somewhere else.

The defense to the center opening is to play in a corner (of which there are four). The only defense to a play in a corner is to play in the center (only one point); the second player has no leeway.

I see now what you mean, you are right! I was under the wrong assumption that first player wins by starting in the center - so Tic-Tac-Toe is harder than I thought :-)

jlt · **#555**

Tic-Tac-Toe is very hard for a go player, because you always try to capture opponent's stones.

Bill Spight · **#556**

jlt wrote:

Tic-Tac-Toe is very hard for a go player, because you always try to capture opponent's stones.

Pente, anyone?

Bill Spight · **#557**

In his new book, Rational Endgame ( viewtopic.php?f=17&t=16567 ), Antti Tormanen does without the terms, sente and gote. (Edit: Correction. Tormanen uses sente but not gote or reverse sente.) Those hoary terms are hoary because they are useful, but, like most words, they also have accrued different meanings, and that can cause confusion. Can we really do without them?

Well, we can. I am reminded of when I first began exploring the endgame. I realized that a theory of the endgame could be built using only final scores. OC, the bots do that, but their theory is based upon errors. Can we do that assuming correct play? Sure. Here is a simple example.

Click Here To Show Diagram Code: [go]$$B Black to play and win $$ . . . X X X X O O O O O O . . $$ . . . X O O b . X X X . O . . $$ . . X X X X O O O O O O O . . $$ . . X O O O a X . O . . . . . $$ . . X . X X X O O O . . . . . $$ . . X X X . . . . . . . . . .[/go]

Play takes place only inside the corridors. All outside stones are alive. Should Black play at "a", or at "b"?

OC, this is easy enough to read out.

Click Here To Show Diagram Code: [go]$$B Black to play and win $$ . . . X X X X O O O O O O . . $$ . . . X O O 2 . X X X . O . . $$ . . X X X X O O O O O O O . . $$ . . X O O O 1 X . O . . . . . $$ . . X . X X X O O O . . . . . $$ . . X X X . . . . . . . . . .[/go]

If Black plays at "a" White plays at "b". White wins by 1 pt.

Click Here To Show Diagram Code: [go]$$B Black to play and win $$ . . . X X X X O O O O O O . . $$ . . . X O O 1 3 X X X . O . . $$ . . X X X X O O O O O O O . . $$ . . X O O O 2 X . O . . . . . $$ . . X . X X X O O O . . . . . $$ . . X X X . . . . . . . . . .[/go]

If Black plays at "b" White plays at "a", and then Black connects to get the last play. Black wins by 1 pt.

So where is the theory? Using CGT notation we can write position "a" as {7 | -3} (ignoring dame). And we can write position "b" as { 4 | -3 || -8}. The theory for a position with these two types of plays is to compare particular score differences for each position. For "a" it is the difference between 7 (after one Black play) and -3 (after one White play), which is 10. For "b" it is the difference between 4 (after two Black plays) and -8 (after one White play), which is 12. 12 > 10, so Black should play at "b". We do not have to worry about whether "b" is sente or not. That does not matter. Suppose that "b" was {8 | -3 || -4}. It would obviously be sente, and since {8 | -3} is hotter than {7 | -3} we should play "b". But that comparison is superfluous, since the rule tells us to play "b", anyway. To read the position out we would have to take {8 | -3} into account, but the rule tells us what to play without having to read the position out. That's what the theory gets us.

Obviously, the theory so far is about comparing these specific types of plays. We can avoid reading the position out, but there will be many rules to memorize. However, these rules will tell us what correct play is.

What about the standard theory? "a" is obviously gote, with a count of 2; each play gains 5 pts. "b" is less obviously gote. It has a count of -3¾; each play gains 4¼ pts. The position after Black plays has a count of +½, and each play gains 3½ pts. Standard theory tells us that we should normally play the move that gains the most. In this case that would be wrong. Standard theory also tells us that getting the last play before a drop in temperature could provide an exception to playing the hottest move. The drop of 3½ pts. is a big clue. But standard theory does not guarantee making the correct play.

Knotwilg · **#558**

Bill, would you mind explaining a few CGT basics underneath this post of yours?

1) And we can write position "b" as { 4 | -3 || -8}.
-- where does the "-3" come from?
2)
The theory for a position with these two types of plays is to compare particular score differences for each position. For "a" it is the difference between 7 (after one Black play) and -3 (after one White play), which is 10. For "b" it is the difference between 4 (after two Black plays) and -8 (after one White play), which is 12. 12 > 10, so Black should play at "b".
-- Why does it not matter here that the "4" is reached after two Black plays?
3)
We do not have to worry about whether "b" is sente or not. That does not matter. Suppose that "b" was {8 | -3 || -4}. It would obviously be sente,
-- Why does it make sense to suppose that "b" was {8 | -3 || -4}?
-- And why is that then obviously sente?

Bill Spight · **#559**

Knotwilg wrote:

Bill, would you mind explaining a few CGT basics underneath this post of yours?

1) And we can write position "b" as { 4 | -3 || -8}.
-- where does the "-3" come from?

After Black plays and White replies, the local score is 4 pts. for Black minus 7 pts. for White, or -3. In { 4 | -3 || -8} 4 is the result after two Black plays, -3 is the result after one Black play and one White reply, and -8 is the result after one White play.

Quote:

2)
The theory for a position with these two types of plays is to compare particular score differences for each position. For "a" it is the difference between 7 (after one Black play) and -3 (after one White play), which is 10. For "b" it is the difference between 4 (after two Black plays) and -8 (after one White play), which is 12. 12 > 10, so Black should play at "b".
-- Why does it not matter here that the "4" is reached after two Black plays?

Oh, it does matter. The theory assumes that if Black plays first in "b" White will reply in "a", and then Black will make a second play in "b". If we rely upon the theory instead of reading the play out, we do not have to check whether White will reply in "b" instead of "a".

Quote:

3)
We do not have to worry about whether "b" is sente or not. That does not matter. Suppose that "b" was {8 | -3 || -4}. It would obviously be sente,
-- Why does it make sense to suppose that "b" was {8 | -3 || -4}?

To show that the theory gives the same answer in that case.

Quote:

-- And why is that then obviously sente?

Because a play in {8 | -3} is bigger than a play in {7 | -3}.

Bill Spight · **#560**

Now let's switch sides.

Click Here To Show Diagram Code: [go]$$W White to play and win $$ . . . X X X X O O O O O . . $$ . . . X O O . X X . X O . . $$ . . X X X X O O O O O O . . $$ . . X O O O . X . O . . . . $$ . . X O X X X O O O . . . . $$ . . X X X . . . . . . . . .[/go]

Click Here To Show Diagram Code: [go]$$W White to play and win $$ . . . X X X X O O O O O . . $$ . . . X O O 1 X X . X O . . $$ . . X X X X O O O O O O . . $$ . . X O O O 2 X . O . . . . $$ . . X O X X X O O O . . . . $$ . . X X X . . . . . . . . . .[/go]

If White plays in "b" Black replies in "a" and wins by 1 pt.

Click Here To Show Diagram Code: [go]$$W White to play and win $$ . . . X X X X O O O O O . . $$ . . . X O O 2 X X 3 X O . . $$ . . X X X X O O O O O O . . $$ . . X O O O 1 X . O . . . . $$ . . X O X X X O O O . . . . $$ . . X X X . . . . . . . . . .[/go]

If White plays in "b" Black replies in "b", then White gets the last play in "b" and wins by 1 pt.

We may write "a" as {8 | -3} and "b" as {4 | 2 || -7}. Since White plays first the relevant score difference in "b" is 2 - ( -7) = 9. That is 2 pts. less than the score difference in "a", so White plays in "a".

Edit: I think it best to continue this on this page.

In this example "a" is a gote with a count of 2½ and each move gains 5½. "b" is also gote with a count of -2 and each play gains 5. The standard theory helps White to make the right choice. However, the comparison is not with 3 - (-7) = 10, where 3 is the count after Black plays, but with 2 - (-7) = 9, where 2 is the score after Black plays and White replies. This theory treats {{ A | B} | C}, where A > B > C, as sente when White is to play and as gote when Black is to play (when the only other play is a single gote). Obviously, with this theory we cannot call that position sente or gote.

This 'n' that

Who is online